The use of granular media filter beds for water filtration is an engrained part of water treatment and under the term ‘granular filter media’ the exclusive use of sand and anthracite is generally assumed. The filter capacity and filtrate quality yielded by different media grain sizes and at different bed depths has been studied extensively. After decades of filtration design and operation many engineers and utilities have determined that one key design criteria is the ratio L/d or bed depth L divided by average filter grain effective size d. The development of high surface area or ‘rough engineered ceramic’ media and the use of improved surface area measurement technologies allows for alternative bed depth to grain size ratios thus altering conventional wisdom regarding L/d ratios. New technology now provides an opportunity to implement shallower beds due to larger effective size media that while having low L/d ratios still provide more overall surface area, better filter performance and better Unit Filter Run Volumes (UFRV) than deeper, finer grain conventional filter beds.
Introduction
General filtration theory maintains that the more filter grains (‘collectors’) you have in a filter or the smaller the filter media average size the better your filtrate quality will be. This phenomenon has been researched for decades, for example Iwasaki (1937) presented a mathematical model that described the removal of particles based on solids influent load, diameter of media and depth of media. According to his equation, as the L/d ratio increases the removal of particle efficiency increases. This theory was then validated by Wegelin et al (1986): L/d ratio—A dimensionless value that is obtained by dividing the length (depth) of a granular media filter bed by the weighted effective diameter‘d’ of the filter media. The weighted effective diameter of the media is calculated based on the percentage of the total bed depth contributed by each media layer.
Effective filtration
Regardless of the filtration theory applied - Brownian motion, inertial impaction, sedimentation, straining or diffusion - the basic truth is that the more media grains and the smaller the grains the greater the effective filtration of particulate. This theory has limitations in relation to both grain size and filter bed depth. When grain size becomes too small the clean filter head loss is impractically high and filter run times are too short, typically due to surface blinding of the fine media. For example, when testing filter sand of 0.5mm size at 24 inch depth (an L/d of 1,220) the clean head loss may be acceptable but the filter surface blinding tendencies may limit the applications for this configuration. When using deep bed filters such as those 6 feet deep with 1.5 mm sand media (an L/d of 1,218), for example, the effluent quality may be similar to that obtained from use of finer filter sand and the clean head loss will be reduced. However, the implementation of beds 6 feet deep has both significant installation and operational costs and may be impractical. For plants using conventional clarification pre-treatment, a common dual filter configuration uses 24 inches of 0.90 mm effective size (ES) anthracite over 12 inches of 0.50 mm ES sand. The L/D ratio (mm depth/mm ES) for the anthracite is 675 and for the sand it is 608. Thus, the summation L/D would be 1283. In general Engineering firms, State Authorities and operators work within the 1000–1200 L/d ratio as a guideline. The L/d ratio value has been derived from years of filtration design and operation. The general consensus is that an L/d ratio of 1200 is the preferred value. It is generally accepted that the coarser top layer will provide interstitial spacing to accommodate larger solids that carry over from coagulation. The selection of the top layer is critical to achieving the Unit Filter Run Volumes (UFRV) thereby providing reasonable filter throughput between backwash events. By utilising the correct grain size at the top section of the filter bed we greatly influence the depth of penetration of solids into the bed. Filtration is all about collector availability and surface area, regardless of the particle attachment phenomenon we embrace. The bottom line is that the amount of available surface area is crucial to the performance of any filter. While the interstitial space sizes, chemical composition of the receptor and receptor shape are all important considerations, the overarching concept is that a minimum amount of surface area is required for effective filtration.
Surface area measurement and evaluation
Surface area measurement of receptors (filter grains) is a rarely discussed topic. Most data regarding surface area presents BET nitrogen sorption (or helium as an alternative gas) and references testing of carbonaceous materials such as anthracite and granular activated carbon (GAC). The use of nitrogen sorption on carbonaceous materials such as anthracite is problematic. The nitrogen molecule is exceedingly small at 3.16 angstroms (0.000316 microns) and will flow into capillaries or micro-fractures within the grain, thus measuring surface area that does not influence particle filtration in any way whatsoever. Pores are classified into three categories according to size: micropores (pore diameter smaller than 2 nm); mesopores (pore diameter 2–50 nm); and macropores (pore diameter larger than 50 nm) [Sing et al. 1985]. Nitrogen gas sorption is ideal for measuring pore diameter ranges from 0.3–300 nm. Mercury porosimetry is best suited for measurement of macropores and surface roughness that range from 300 nm–200 microns). Testing of pores smaller than 300 nm requires mercury pressures applied in excess of 60,000 psi which results in a significant amount of particle distortion and significant data errors. The measurement of pores in the 0.5 micron range requires an applied mercury pressure of ~450 psi and at this pressure particle compression and distortion is minimised. According to Mahajan (1991): On the whole, there is wide acceptance of the following general model for the porous structure of coal; that coals contain an interconnected pore network of high surface area, the slit-shaped pores having constricted openings of molecular dimensions...[and] coals contain both open and closed porosity.
Differences in total surface area
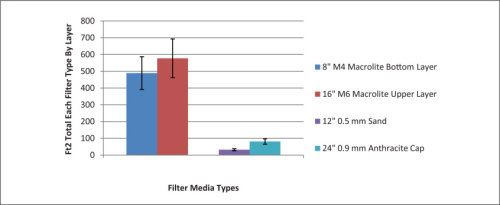
Gan et al. (1972) tested both nitrogen, helium and mercury intrusion on anthracite coal and discovered significant differences in total surface area depending on the test method. Three different surface area test procedures would identify the total surface area by pore type: • Mercury intrusion measuring pores >300 Å making up 11.9% of the total surface area. • Nitrogen BET measuring pores 12–300 Å making up 13.1%. • Helium BET measuring pores < 12 Å making up 75% of the measurable surface area. For the purposes of particle filtration in conventional applications, the surface irregularities below 500 nanometres should be disregarded. Impressions or ‘pores’ below 0.5 microns will be covered over by larger individual or agglomerated particles and therefore are of little use unless the influent water contains sub-micron colloids which is not the norm. Table 1 shows the results of mercury intrusion testing of common filtration materials as well as the rough engineered ceramic media Macrolite. The test data has been focused on pores that are ≥ 0.5 microns in size and measure surface impressions up to ≤ 250 microns in size. Limiting the upper spectrum size to 250 microns helps eliminate errors caused at low applied pressures where the mercury is simply filling grain to grain interstitial spaces. Figure 4 shows the total surface area contribution by layer for the two types of filter configurations.
Conclusions
Surface area analysis clearly shows that by using rough engineered ceramic filter media a comparatively short bed height can have substantially higher overall surface area compared to conventional media. While surface area is a primary influence on filtration performance it is not by any means the only influencing factor. When implementing rough engineered ceramic media the overall filter bed surface area can be increased by orders of magnitude compared to sand and anthracite. However, the reduction in bed height when using rough engineered ceramics is not proportional to the increased surface area.
| Filter material by type and size | Surface area of pores larger than 0.5 microns (M2/cc) | Surface area of pores larger than 0.5 microns (M2/M3) | Surface area of pores larger than 0.5 microns (ft2/ft3) |
| M4 Macrolite 0.55 mm ES | 0.329 | 329, 179.0 | 866.0 |
| M6 Macrolite 1.2 mm ES | 0.139 | 139, 278.0 | 366.0 |
| Sand 2040 0.5 mm ES | 0.0121 | 12, 164.0 | 32.0 |
| Anthracite 0.9 mm ES | 0.0154 | 15, 404.0 | 41.0 |
Filters require a minimum number of filter grain collectors in the path of filtrate. The number of collectors influences the percentage chance of particle interception. Therefore, a filter bed with too short an overall height will struggle to meet quality expectations. In addition, the surface roughness of engineered media will undoubtedly have different hydrodynamic forces on the filtrate due to the surface irregularities and these forces may or may not improve filter performance. The combination of roughness and the subsequent hydrodynamic influences may also allow for increased flow per unit area (gpm/ft2) filter flow rates. The validation for switching to a media such as Macrolite should involve field evaluations in the form of filtration pilots. A properly designed filter pilot is a useful tool in evaluating the full scale filter design and confirming the optimal filter bed height.



